Đề thi Violympic Toán Tiếng Anh lớp 8 vòng 10 năm 2015 - 2016

Đề thi Violympic Toán Tiếng Anh lớp 8 vòng 10

Đề thi Violympic Toán Tiếng Anh lớp 8 vòng 10 năm 2015 - 2016 là đề thi giải Toán bằng Tiếng Anh qua mạng dành cho học sinh lớp 8 có đáp án đi cùng được VnDoc sưu tầm và giới thiệu tới các em học sinh hi vọng giúp các em ôn tập và củng cố kiến thức, nhằm đạt kết quả cao trong các vòng tiếp theo của cuộc thi Violympic giải Toán Tiếng Anh qua mạng năm học 2016 - 2017.

Đề thi Violympic Toán Tiếng Anh lớp 8 vòng 10 năm 2015 - 2016 trực tuyến

Exam number 1: Đỉnh núi trí tuệ

Question 1: If a + b = 3, a² + b² = 7 then a³ + b³ = ..........

Question 2: Find the value of k such that x³ + kx² + (4 - k)x - 35 is divisible by x - 7.

Question 3: An isosceles trapezoid ABCD is shown in the following diagram. What is the area of the trapezoid ABCD?

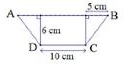
Figure is not drawn to scale.

Question 4: Find the value of x such that: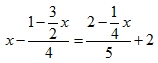

Question 5: Let ABCD be a trapezoid with AB // CD, Â = D = 90^o and AB = AD = CD/2. Find the measure of the angle BCD.

Question 6: Let ABCD.A'B'C'D' be a cube with AC' = √3cm. Find the total surface area of this cube.

Question 7: Bottle A contains 15% syrup. Bottle B contains 40% syrup. When these 2 bottles of syrup are mixed, the syrup content is 30% and the total volume is 600ml. How much syrup is in the bottle A at first?

Question 8: Let ABC be a triangle with AB = 3cm, AC = 7cm. The internal bisector of the angle BAC intersects BC at D. The line passing through D and parallel to AC cuts AB at E. Find the measure of DE.

Question 9: If x - y - z = 0 and x + 2y - 10z = 0, z ≠ 0 then the value of 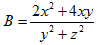is ............

Question 10: Given the equation (x - m)(m - 1) + (x - 1)(m + 1) = -2m. Find all values of m such that this equation has no solution.

Question 11: Let ABCD be a trapezoid with bases AB, CD and O be the intersection of AC and BD. If the areas of triangle OAB, triangle OCD are 16cm², 40cm² respectively and M is the midpoint of BD, then the area of the triangle AMD is .........cm².

Question 12: Given the equation:

The average (arithmetic mean) of all roots of this equation is ........... Write your answer by fraction in simplest form

Question 13: The Ford car left Hanoi for Nghe An and the Audi car left Nghe An for Ha Noi at the same time. The ratio of their speeds (the Ford car to the Audi car) was 4 : 3. The Ford decreased its speed by 25% and the Audi car increased its speed by 25% after they had passed each other.

When the Ford car reached Nghe An, the Audi car was still 20km away from Hanoi. The distance between Hanoi and Nghe An is ...... km.

Question 14: Suppose that the polynomial f(x) = x⁵ - x⁴ - 4x³ + 2x² + 4x + 1 has 5 solutions x₁; x₂; x₃; x₄; x₅. The other polynomial k(x) = x² - 4. Find the value of P = k(x₁) x k(x₂) x k(x₃) x k(x₄) x k(x₅)

Question 15: The smallest value of 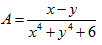is ............. Write your answer by decimal in simplest form

Exam number 2: Cóc vàng tài ba

Question 1: Which one could be the next?

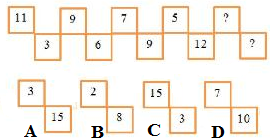
A. Đáp án A

B. Đáp án B

C. Đáp án C

D. Đáp án D

Question 2: Assume that two numbers x and y satisfy: 2x + y = 6. Find the minimum value of expression A = 4x² + y²

A. 18 B. 36 C. 6 D. 24

Question 3: ABCD is a square of side length 5cm. DEFG is a square of side length 3cm such that E lies on the extension of CD and G lies on AD. Find the area, in cm², of triangle BDF.

A. 15 B. 25 C. 20 D. 17

Question 4: In the xy - plane, given three points A(-1; 2); B(-3; -1); D(6; 2). If ABCD is a parallelogram then C = ........

A. (-4; 1) B. (4; -1) C. (-4; 1) D. (-4; -1)

Question 5: If a and b are two non-zero distinct numbers such that 3a² + 4b² = 7ab then the value of the expression 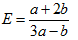is ...........

A. 7/3 B. 3/2 C. 7/4 D. 10/9

Question 6: Given the rectangle whose perimeter is 24cm. If its length is decreased by 1cm and its width is increased by 1cm, then the area of the original rectanle is increased by 3cm². Find the area of the original rectangle.

A. 32cm² B. 35cm² C. 40cm² D. 48cm²

Question 7: The triangle ABC has AB = 5cm, AC = 8cm, Â = 60o and the internal bisector AD (D ∈ BC). The length of BD is .........cm.

A. 35/13 B. 40/13 C. 20/7 D. 13/40

Question 8: What number should replace the question mark?

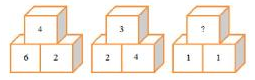
A. 0 B. 2 C. 1 D. 3
Question 9: If all roots of the polynomial P(x) = x² + 5x - 1 are also roots of the polynomial Q(x) = x³ + ax² + bx + c then the value of a + b + 6c is ............

A. 4 B. 8 C. -4 D. -5

Question 10: Let ABC be an isoceles triangle (AB = AC) and its area is 501cm². BD is the internal bisector of the angle ABC (D ∈ AC), E is a point on the opposite ray of CA such that CE = CB. I is a point on BC such that CI = 1/2 BI. The line EI meets AB at K, BD meets KC at H. Find the area of the triangle AHC.

A. 167cm² B. 250,5cm² C. 200cm² D. 176cm²

Exam number 3: Điền giá trị thích hợp vào chỗ chấm

Question 1: The number of roots of the equation Ιx3 - 8Ι = 16 - 2x³ is .........

Question 2: Find the greatest interger number x such that the value of (3x - 2)/4 is greater than the value of (5x + 3)/5.
Answer: The greatest integer number x is .........

Question 3: How many sides does a polygon have if the number of its diagonals is as triple as the number of its sides?

Question 4: Find the positive value of k such that x = 2 is a root of the following equation: x² - kx + k² - 4 = 0

Question 5: If x, y, z satisfy these equations yz = 3/2 - x²/2; zx = 1/2 - y²/2 and xy = 5/2 - z²/2 then the value of Ιx + y + zΙ is ...........
Question 6: Find the remainder when (x + 2)(x + 3)(x + 4)(x + 5) + 2017 is divided by x2 + 7x + 11.

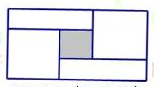
Question 7: A rectangle has a length of 60cm and a width of 30cm. It is cut into 2 indentical squares, 2 identical rectangles and a shaded small square. Find the area of the shaded square. Find the area of the shaded square.

Question 8: Given P(x) = (x² - 1/2 x - 1/2)¹⁰⁰⁸

If P(x) = a₂₀₁₆x²⁰¹⁶ + a₂₀₁₅x²⁰¹⁵ + ..... + a₁x + a₀ then the value of the sum a₀ + a₂ + a₄ + .... + a₂₀₁₄ is ........... Write your answer by decimal in simplest form

Question 9: The number of ordered pairs (x; y) where x, y ∈ N* such that x²y² - 2(x + y) is perfect square is ...........

Question 10: Let ABCD be the square with the side length 56cm. If E and F lie on CD, C respectively such that CF = 14cm and EAF = 45o then CE = ........cm.

Đáp án đề thi Violympic Toán Tiếng Anh lớp 8

Exam number 1: Đỉnh núi trí tuệ

Question 1: 18

Question 2: -8

Question 3: 90

Question 4: 106/57

Question 5: 45

Question 6: 6

Question 7: 240

Question 8: 21/10

Question 9: 8

Question 10: 0

Question 11: 22

Question 12: 9/4

Question 13: 560

Question 14: -15

Question 15: -0,25

Exam number 2: Cóc vàng tài ba

Question 1: A

Question 2: A

Question 3: A

Question 4: B

Question 5: D

Question 6: A

Question 7: A

Question 8: C

Question 9: A

Question 10: A

Exam number 3: Hãy điền giá trị thích hợp vào chỗ chấm.

Question 1: 1

Question 2: -5

Question 3: 9

Question 4: 2

Question 5: 3

Question 6: 2016

Question 7: 225

Question 8: -0,5

Question 9: 2

Question 10: 48