Giao diện mới của VnDoc Pro: Dễ sử dụng hơn - chỉ tập trung vào lớp bạn quan tâm. Vui lòng chọn lớp mà bạn quan tâm: Lưu và trải nghiệm

VnDoc.com

Bộ 12 đề thi giữa kì 1 Toán 9 năm 2023 - 2024 có đáp án

Đề kiểm tra giữa kì 1 Toán 9

Đề kiểm tra giữa kì 1 Toán 9 có đáp án

Đề kiểm tra giữa kì 1 Toán 9 – Đề số 1
Đáp án đề thi giữa học kì 1 Toán 9 – Đề số 1
Đề thi giữa học kì 1 Toán 9 – Đề số 2
Đáp án đề kiểm tra giữa kì 1 Toán 9 – Đề số 2

Bộ đề thi giữa kì 1 Toán 9 năm 2023 - 2024 là bộ đề thi mới nhất nằm trong chương trình kiểm tra giữa học kì 1 lớp 9. Bộ đề Toán giữa kì 1 bao gồm 12 đề kiểm tra khác nhau, sẽ giúp ích cho các em học sinh ôn tập, rèn luyện kĩ năng giải đề thi biết cách phân bổ thời gian hợp lý. Mời các bạn tải về tham khảo chi tiết.

Đề kiểm tra giữa kì 1 Toán 9 – Đề số 1

Bài 1 (3 điểm): Cho biểu thức:

$P = \frac{{\sqrt x + 2}}{{\sqrt x - 3}} + \frac{{2\sqrt x }}{{\sqrt x + 3}} + \frac{{3x - 8\sqrt x + 27}}{{9 - x}}$ $P = \frac{{\sqrt x + 2}}{{\sqrt x - 3}} + \frac{{2\sqrt x }}{{\sqrt x + 3}} + \frac{{3x - 8\sqrt x + 27}}{{9 - x}}$

a) Tìm điều kiện của x để biểu thức P có nghĩa?

b) Rút gọn biểu thức

c) Tìm giá trị x nguyên để P nhận giá trị nguyên

Bài 2 (2 điểm): Tính giá trị của biểu thức:

a) $A = \sqrt {13 - 4\sqrt 3 } + \sqrt {13 + 4\sqrt 3 }$ $A = \sqrt {13 - 4\sqrt 3 } + \sqrt {13 + 4\sqrt 3 }$

b) $B = \frac{{2\sqrt 3 - 3\sqrt 2 }}{{\sqrt 6 }} + \frac{1}{{\sqrt 2 + \sqrt 3 }}$ $B = \frac{{2\sqrt 3 - 3\sqrt 2 }}{{\sqrt 6 }} + \frac{1}{{\sqrt 2 + \sqrt 3 }}$

Bài 3 (2 điểm): Giải phương trình:

a) ${x^2} - 4x - 45 = 0$ ${x^2} - 4x - 45 = 0$

b) $\frac{{3\sqrt x + 1}}{{x - 9}} = \frac{2}{{\sqrt x - 3}} - \frac{{\sqrt x - 3}}{{\sqrt x + 3}}$ $\frac{{3\sqrt x + 1}}{{x - 9}} = \frac{2}{{\sqrt x - 3}} - \frac{{\sqrt x - 3}}{{\sqrt x + 3}}$

Bài 4 (3 điểm): Cho tam giác ABC (AB < AC) có $\widehat B + \widehat C = {90^0}$ $\widehat B + \widehat C = {90^0}$, AH là đường cao xuất phát từ đỉnh A (H ∈ BC). Gọi D là hình chiếu của H lên AB (D ∈ AB) và E là hình chiếu của H lên AC (E ∈ AC).

a) Chứng minh ADHE là hình chữ nhật

b) Chứng minh AD.AB = AE.AC

c) Biết AB = 6cm và AC = 8cm. Tính độ dài BC, AH, AD và AE

Đáp án đề thi giữa học kì 1 Toán 9 – Đề số 1

Bài 1:

a) Để P có nghĩa _{$\Leftrightarrow \left\{ \begin{array}{l} x \ge 0\\ x \ne 9 \end{array} \right.$}

b) $P = \frac{{\sqrt x + 2}}{{\sqrt x - 3}} + \frac{{2\sqrt x }}{{\sqrt x + 3}} + \frac{{3x - 8\sqrt x + 27}}{{9 - x}}$ $P = \frac{{\sqrt x + 2}}{{\sqrt x - 3}} + \frac{{2\sqrt x }}{{\sqrt x + 3}} + \frac{{3x - 8\sqrt x + 27}}{{9 - x}}$

$P = \frac{{\left( {\sqrt x + 2} \right)\left( {\sqrt x + 3} \right)}}{{\left( {\sqrt x - 3} \right)\left( {\sqrt x + 3} \right)}} + \frac{{2\sqrt x \left( {\sqrt x - 3} \right)}}{{\left( {\sqrt x - 3} \right)\left( {\sqrt x + 3} \right)}} - \frac{{3x - 8\sqrt x + 27}}{{\left( {\sqrt x - 3} \right)\left( {\sqrt x + 3} \right)}}$ $P = \frac{{\left( {\sqrt x + 2} \right)\left( {\sqrt x + 3} \right)}}{{\left( {\sqrt x - 3} \right)\left( {\sqrt x + 3} \right)}} + \frac{{2\sqrt x \left( {\sqrt x - 3} \right)}}{{\left( {\sqrt x - 3} \right)\left( {\sqrt x + 3} \right)}} - \frac{{3x - 8\sqrt x + 27}}{{\left( {\sqrt x - 3} \right)\left( {\sqrt x + 3} \right)}}$

$P = \frac{{x + 3\sqrt x + 2\sqrt x + 6 + 2x - 6\sqrt x - \left( {3x - 8\sqrt x + 27} \right)}}{{\left( {\sqrt x - 3} \right)\left( {\sqrt x + 3} \right)}}$ $P = \frac{{x + 3\sqrt x + 2\sqrt x + 6 + 2x - 6\sqrt x - \left( {3x - 8\sqrt x + 27} \right)}}{{\left( {\sqrt x - 3} \right)\left( {\sqrt x + 3} \right)}}$

$P = \frac{{7\sqrt x - 21}}{{\left( {\sqrt x - 3} \right)\left( {\sqrt x + 3} \right)}} = \frac{{7\left( {\sqrt x - 3} \right)}}{{\left( {\sqrt x - 3} \right)\left( {\sqrt x + 3} \right)}} = \frac{7}{{\sqrt x + 3}}$ $P = \frac{{7\sqrt x - 21}}{{\left( {\sqrt x - 3} \right)\left( {\sqrt x + 3} \right)}} = \frac{{7\left( {\sqrt x - 3} \right)}}{{\left( {\sqrt x - 3} \right)\left( {\sqrt x + 3} \right)}} = \frac{7}{{\sqrt x + 3}}$

Vậy $P = \frac{7}{{\sqrt x + 3}}$ $P = \frac{7}{{\sqrt x + 3}}$

c) Để P nhận giá trị nguyên

Ta có bảng:

$\sqrt x + 3$ $\sqrt x + 3$	- 7	- 1	1	7
$\sqrt x$ $\sqrt x$	- 10 (loại)	- 4 (loại)	- 2 (loại)	4
x				16 (tm)

Vậy để P nhận giá trị nguyên thì x = 16.

Bài 2:

a) $A = \sqrt {13 - 4\sqrt 3 } + \sqrt {13 + 4\sqrt 3 }$ $A = \sqrt {13 - 4\sqrt 3 } + \sqrt {13 + 4\sqrt 3 }$

$A = \sqrt {1 - 2.1.2\sqrt 3 + 12} + \sqrt {1 + 2.1.2\sqrt 3 + 12}$ $A = \sqrt {1 - 2.1.2\sqrt 3 + 12} + \sqrt {1 + 2.1.2\sqrt 3 + 12}$

$A = \sqrt {{{\left( {1 - 2\sqrt 3 } \right)}^2}} + \sqrt {{{\left( {1 + 2\sqrt 3 } \right)}^2}}$ $A = \sqrt {{{\left( {1 - 2\sqrt 3 } \right)}^2}} + \sqrt {{{\left( {1 + 2\sqrt 3 } \right)}^2}}$

$A = 2\sqrt 3 - 1 + 1 + 2\sqrt 3 = 4\sqrt 3$ $A = 2\sqrt 3 - 1 + 1 + 2\sqrt 3 = 4\sqrt 3$

b) $B = \frac{{2\sqrt 3 - 3\sqrt 2 }}{{\sqrt 6 }} + \frac{1}{{\sqrt 2 + \sqrt 3 }}$ $B = \frac{{2\sqrt 3 - 3\sqrt 2 }}{{\sqrt 6 }} + \frac{1}{{\sqrt 2 + \sqrt 3 }}$

$B = \frac{{\sqrt 6 \left( {\sqrt 2 - \sqrt 3 } \right)}}{{\sqrt 6 }} + \frac{1}{{\sqrt 2 + \sqrt 3 }}$ $B = \frac{{\sqrt 6 \left( {\sqrt 2 - \sqrt 3 } \right)}}{{\sqrt 6 }} + \frac{1}{{\sqrt 2 + \sqrt 3 }}$

$B = \sqrt 2 - \sqrt 3 + \frac{1}{{\sqrt 2 + \sqrt 3 }}$ $B = \sqrt 2 - \sqrt 3 + \frac{1}{{\sqrt 2 + \sqrt 3 }}$

$B = \frac{{\left( {\sqrt 2 - \sqrt 3 } \right)\left( {\sqrt 2 + \sqrt 3 } \right) + 1}}{{\sqrt 2 + \sqrt 3 }}$ $B = \frac{{\left( {\sqrt 2 - \sqrt 3 } \right)\left( {\sqrt 2 + \sqrt 3 } \right) + 1}}{{\sqrt 2 + \sqrt 3 }}$

$B = \frac{{\left( {2 - 3} \right) + 1}}{{\sqrt 2 + \sqrt 3 }} = \frac{{ - 1 + 1}}{{\sqrt 2 + \sqrt 3 }} = \frac{0}{{\sqrt 2 + \sqrt 3 }} = 0$ $B = \frac{{\left( {2 - 3} \right) + 1}}{{\sqrt 2 + \sqrt 3 }} = \frac{{ - 1 + 1}}{{\sqrt 2 + \sqrt 3 }} = \frac{0}{{\sqrt 2 + \sqrt 3 }} = 0$

Bài 3:

a) ${x^2} - 4x - 45 = 0$ ${x^2} - 4x - 45 = 0$

$\Leftrightarrow {x^2} - 9x + 5x - 45 = 0$ $\Leftrightarrow {x^2} - 9x + 5x - 45 = 0$

$\Leftrightarrow x\left( {x - 9} \right) + 5\left( {x - 9} \right) = 0$ $\Leftrightarrow x\left( {x - 9} \right) + 5\left( {x - 9} \right) = 0$

$\Leftrightarrow \left( {x + 5} \right)\left( {x - 9} \right) = 0 \Leftrightarrow \left[ \begin{array}{l} x + 5 = 0\\ x - 9 = 0 \end{array} \right. \Leftrightarrow \left[ \begin{array}{l} x = - 5\\ x = 9 \end{array} \right.$ $\Leftrightarrow \left( {x + 5} \right)\left( {x - 9} \right) = 0 \Leftrightarrow \left[ \begin{array}{l} x + 5 = 0\\ x - 9 = 0 \end{array} \right. \Leftrightarrow \left[ \begin{array}{l} x = - 5\\ x = 9 \end{array} \right.$

Vậy S = {-5; 9}

b) $\frac{{3\sqrt x + 1}}{{x - 9}} = \frac{2}{{\sqrt x - 3}} - \frac{{\sqrt x - 3}}{{\sqrt x + 3}}\left( {x \ge 0;x \ne 9} \right)$ $\frac{{3\sqrt x + 1}}{{x - 9}} = \frac{2}{{\sqrt x - 3}} - \frac{{\sqrt x - 3}}{{\sqrt x + 3}}\left( {x \ge 0;x \ne 9} \right)$

$\Leftrightarrow 3\sqrt x + 1 = 2\left( {\sqrt x + 3} \right) - \left( {\sqrt x - 3} \right)\left( {\sqrt x - 3} \right)$ $\Leftrightarrow 3\sqrt x + 1 = 2\left( {\sqrt x + 3} \right) - \left( {\sqrt x - 3} \right)\left( {\sqrt x - 3} \right)$

$\Leftrightarrow 3\sqrt x + 1 = 2\sqrt x + 6 - \left( {x - 6\sqrt x + 9} \right)$ $\Leftrightarrow 3\sqrt x + 1 = 2\sqrt x + 6 - \left( {x - 6\sqrt x + 9} \right)$

$\Leftrightarrow 3\sqrt x + 1 = 2\sqrt x + 6 - x + 6\sqrt x - 9$ $\Leftrightarrow 3\sqrt x + 1 = 2\sqrt x + 6 - x + 6\sqrt x - 9$

$\Leftrightarrow x - 5\sqrt x + 4 = 0$ $\Leftrightarrow x - 5\sqrt x + 4 = 0$

$\Leftrightarrow x - \sqrt x - 4\sqrt x + 4 = 0$ $\Leftrightarrow x - \sqrt x - 4\sqrt x + 4 = 0$

$\Leftrightarrow \sqrt x \left( {\sqrt x - 1} \right) - 4\left( {\sqrt x - 1} \right) = 0$ $\Leftrightarrow \sqrt x \left( {\sqrt x - 1} \right) - 4\left( {\sqrt x - 1} \right) = 0$

$\Leftrightarrow \left( {\sqrt x - 4} \right)\left( {\sqrt x - 1} \right) = 0 \Leftrightarrow \left[ \begin{array}{l} \sqrt x = 4\\ \sqrt x = 1 \end{array} \right. \Leftrightarrow \left[ \begin{array}{l} x = 16\\ x = 1 \end{array} \right.\left( {tm} \right)$ $\Leftrightarrow \left( {\sqrt x - 4} \right)\left( {\sqrt x - 1} \right) = 0 \Leftrightarrow \left[ \begin{array}{l} \sqrt x = 4\\ \sqrt x = 1 \end{array} \right. \Leftrightarrow \left[ \begin{array}{l} x = 16\\ x = 1 \end{array} \right.\left( {tm} \right)$

Vậy S = {1; 16}

Bài 4:

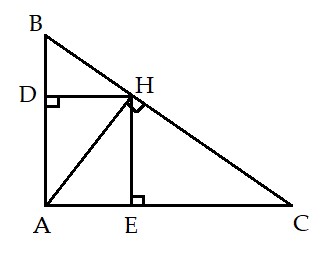
Đề thi giữa học kì 1 Toán 9 năm học 2020 - 2021 - Đề 1

a) + Xét tam giác ABC có: $\widehat A + \widehat B + \widehat C = {180^0}$ $\widehat A + \widehat B + \widehat C = {180^0}$(tổng ba góc trong tam giác)

mà $\widehat B + \widehat C = {90^0} \Rightarrow \widehat A = {90^0}$ $\widehat B + \widehat C = {90^0} \Rightarrow \widehat A = {90^0}$

+ Xét tứ giác ADHE có:

$\widehat {DAE} = {90^0}$ $\widehat {DAE} = {90^0}$(cmt)

$\widehat {HDA} = {90^0}$ $\widehat {HDA} = {90^0}$( $HD \bot AB$ $HD \bot AB$ - gt)

$\widehat {HEA} = {90^0}$ $\widehat {HEA} = {90^0}$( $HE \bot AC$ $HE \bot AC$ - gt)

$\Rightarrow$ $\Rightarrow$ADHE là hình chữ nhật (dhnb) (đpcm)

b) + Xét tam giác ABH có $\widehat {AHB} = {90^0};HD \bot AB$ $\widehat {AHB} = {90^0};HD \bot AB$:

$A{H^2} = AD.AB$ $A{H^2} = AD.AB$(hệ thức lượng trong tam giác vuông) (1)

+ Xét tam giác AHC có $\widehat {AHC} = {90^0};HE \bot AC$ $\widehat {AHC} = {90^0};HE \bot AC$:

$A{H^2} = AE.AC$ $A{H^2} = AE.AC$(hệ thức lượng trong tam giác vuông) (2)

+ Từ (1) và (2) $\Rightarrow AD.AB = AE.AC\left( { = A{H^2}} \right)$ $\Rightarrow AD.AB = AE.AC\left( { = A{H^2}} \right)$(đpcm)

c) + Xét tam giác ABC có $\widehat {BAC} = {90^0};AH \bot BC$ $\widehat {BAC} = {90^0};AH \bot BC$:

$A{B^2} + A{C^2} = B{C^2}$ $A{B^2} + A{C^2} = B{C^2}$(Pitago)

$\Rightarrow BC = \sqrt {A{B^2} + A{C^2}} = \sqrt {100} = 10$ $\Rightarrow BC = \sqrt {A{B^2} + A{C^2}} = \sqrt {100} = 10$(cm)

$\frac{1}{{A{H^2}}} = \frac{1}{{A{B^2}}} + \frac{1}{{A{C^2}}}$ $\frac{1}{{A{H^2}}} = \frac{1}{{A{B^2}}} + \frac{1}{{A{C^2}}}$(hệ thức lượng trong tam giác vuông)

$\Rightarrow AH = \sqrt {\frac{{A{B^2}.A{C^2}}}{{A{B^2} + A{C^2}}}} = \frac{{24}}{5}$ $\Rightarrow AH = \sqrt {\frac{{A{B^2}.A{C^2}}}{{A{B^2} + A{C^2}}}} = \frac{{24}}{5}$(cm)

+ Từ (1) $\Rightarrow AD = \frac{{A{H^2}}}{{AB}} = \frac{{96}}{{25}}$ $\Rightarrow AD = \frac{{A{H^2}}}{{AB}} = \frac{{96}}{{25}}$(cm)

+ Từ (2) $\Rightarrow AE = \frac{{A{H^2}}}{{AC}} = \frac{{72}}{{25}}$ $\Rightarrow AE = \frac{{A{H^2}}}{{AC}} = \frac{{72}}{{25}}$(cm)

Đề thi giữa học kì 1 Toán 9 – Đề số 2

Bài 1 (1 điểm): Tìm điều kiện để các căn thức dưới đây có nghĩa:

a) $\sqrt {16 - 4x}$ $\sqrt {16 - 4x}$

b) $\sqrt {3x + 7}$ $\sqrt {3x + 7}$

Bài 2 (2 điểm): Rút gọn các biểu thức dưới đây:

a) $A = \sqrt {72} - \sqrt 4 .\frac{1}{2} + \sqrt {32} + \sqrt {162}$ $A = \sqrt {72} - \sqrt 4 .\frac{1}{2} + \sqrt {32} + \sqrt {162}$

b) $B = \frac{1}{{\sqrt 7 - 4}} + \frac{1}{{\sqrt 7 + 4}}$ $B = \frac{1}{{\sqrt 7 - 4}} + \frac{1}{{\sqrt 7 + 4}}$

Bài 3 (2 điểm): Cho hai biểu thức $M = \frac{1}{{\sqrt x - \sqrt {x - 1} }} - \frac{1}{{\sqrt x + \sqrt {x - 1} }}$ $M = \frac{1}{{\sqrt x - \sqrt {x - 1} }} - \frac{1}{{\sqrt x + \sqrt {x - 1} }}$và $N = \frac{{\sqrt {x - 1} }}{{\sqrt x - 5}}$ $N = \frac{{\sqrt {x - 1} }}{{\sqrt x - 5}}$

a) Rút gọn biểu thức P = M:N

b) Tính giá trị của biểu thức P tại $x = 4 - 2\sqrt 3$ $x = 4 - 2\sqrt 3$

Bài 4 (2 điểm): Giải phương trình:

a) ${x^2} - 8x - 9 = 0$ ${x^2} - 8x - 9 = 0$

b) $\sqrt {5x + 4} = x + 2$ $\sqrt {5x + 4} = x + 2$

Bài 5 (3 điểm): Cho tam giác ABC có AB = 6cm, AC = 8cm, BC = 10cm.

a) Chứng minh tam giác ABC là tam giác vuông.

b) Vẽ đường cao AH (H ∈ BC). Tính độ dài của BH, HC và AH.

c) Trên tia đối của tia BA, lấy điểm D sao cho BD = BC. Chứng minh: ${\mathop{\rm AD}\nolimits} .BC = \frac{{{{{\mathop{\rm CD}\nolimits} }^2}}}{2}$ ${\mathop{\rm AD}\nolimits} .BC = \frac{{{{{\mathop{\rm CD}\nolimits} }^2}}}{2}$

d) Tính diện tích tam giác BCD

Đáp án đề kiểm tra giữa kì 1 Toán 9 – Đề số 2

Bài 1:

a) Để biểu thức $\sqrt {16 - 4x}$ $\sqrt {16 - 4x}$ có nghĩa thì $16 - 4x \ge 0 \Leftrightarrow x \le 4$ $16 - 4x \ge 0 \Leftrightarrow x \le 4$

b) Để biểu thức $\sqrt {3x + 7}$ $\sqrt {3x + 7}$ có nghĩa thì $3x + 7 \ge 0 \Leftrightarrow x \ge \frac{{ - 7}}{3}$ $3x + 7 \ge 0 \Leftrightarrow x \ge \frac{{ - 7}}{3}$

Bài 2:

a) $A = \sqrt {72} - \sqrt 4 .\frac{1}{2} + \sqrt {32} + \sqrt {162}$ $A = \sqrt {72} - \sqrt 4 .\frac{1}{2} + \sqrt {32} + \sqrt {162}$

$A = \sqrt {36.2} - 2.\frac{1}{2} + \sqrt {16.2} + \sqrt {81.2}$ $A = \sqrt {36.2} - 2.\frac{1}{2} + \sqrt {16.2} + \sqrt {81.2}$

$A = 6\sqrt 2 - 1 + 4\sqrt 2 + 9\sqrt 2$ $A = 6\sqrt 2 - 1 + 4\sqrt 2 + 9\sqrt 2$

$A = 19\sqrt 2 - 1$ $A = 19\sqrt 2 - 1$

b) $B = \frac{1}{{\sqrt 7 - 4}} + \frac{1}{{\sqrt 7 + 4}} = \frac{{\sqrt 7 + 4 + \sqrt 7 - 4}}{{\left( {\sqrt 7 - 4} \right)\left( {\sqrt 7 + 4} \right)}} = \frac{{2\sqrt 7 }}{{7 - 16}} = \frac{{2\sqrt 7 }}{{ - 9}} = \frac{{ - 2\sqrt 7 }}{9}$ $B = \frac{1}{{\sqrt 7 - 4}} + \frac{1}{{\sqrt 7 + 4}} = \frac{{\sqrt 7 + 4 + \sqrt 7 - 4}}{{\left( {\sqrt 7 - 4} \right)\left( {\sqrt 7 + 4} \right)}} = \frac{{2\sqrt 7 }}{{7 - 16}} = \frac{{2\sqrt 7 }}{{ - 9}} = \frac{{ - 2\sqrt 7 }}{9}$

Bài 3:

a) $M = \frac{1}{{\sqrt x - \sqrt {x - 1} }} - \frac{1}{{\sqrt x + \sqrt {x - 1} }}$ $M = \frac{1}{{\sqrt x - \sqrt {x - 1} }} - \frac{1}{{\sqrt x + \sqrt {x - 1} }}$; điều kiện $x \ge 1$ $x \ge 1$

$M = \frac{{\sqrt x + \sqrt {x - 1} - \left( {\sqrt x - \sqrt {x - 1} } \right)}}{{\left( {\sqrt x - \sqrt {x - 1} } \right)\left( {\sqrt x + \sqrt {x - 1} } \right)}} = \frac{{2\sqrt {x - 1} }}{{x - \left( {x - 1} \right)}} = 2\sqrt {x - 1}$ $M = \frac{{\sqrt x + \sqrt {x - 1} - \left( {\sqrt x - \sqrt {x - 1} } \right)}}{{\left( {\sqrt x - \sqrt {x - 1} } \right)\left( {\sqrt x + \sqrt {x - 1} } \right)}} = \frac{{2\sqrt {x - 1} }}{{x - \left( {x - 1} \right)}} = 2\sqrt {x - 1}$

$N = \frac{{\sqrt {x - 1} }}{{\sqrt x - 5}}$ $N = \frac{{\sqrt {x - 1} }}{{\sqrt x - 5}}$; điều kiện $x \ge 0;x \ne 25$ $x \ge 0;x \ne 25$

$P = M:N = 2\sqrt {x - 1} .\frac{{\sqrt x - 5}}{{\sqrt {x - 1} }} = 2\left( {\sqrt x - 5} \right)$ $P = M:N = 2\sqrt {x - 1} .\frac{{\sqrt x - 5}}{{\sqrt {x - 1} }} = 2\left( {\sqrt x - 5} \right)$

Vậy $P = 2\left( {\sqrt x - 5} \right)$ $P = 2\left( {\sqrt x - 5} \right)$

b) Tại $x = 4 - 2\sqrt 3$ $x = 4 - 2\sqrt 3$(tm) thì $\sqrt x = \sqrt {4 - 2\sqrt 3 } = \sqrt {{{\left( {\sqrt 3 - 1} \right)}^2}} = \sqrt 3 - 1$ $\sqrt x = \sqrt {4 - 2\sqrt 3 } = \sqrt {{{\left( {\sqrt 3 - 1} \right)}^2}} = \sqrt 3 - 1$

Có $P = 2\left( {\sqrt 3 - 1 - 5} \right) = 2\left( {\sqrt 3 - 6} \right) = 2\sqrt 3 - 12$ $P = 2\left( {\sqrt 3 - 1 - 5} \right) = 2\left( {\sqrt 3 - 6} \right) = 2\sqrt 3 - 12$

Vậy tại $x = 4 - 2\sqrt 3$ $x = 4 - 2\sqrt 3$ thì $P = 2\sqrt 3 - 12$ $P = 2\sqrt 3 - 12$

Bài 4:

a) ${x^2} - 8x - 9 = 0$ ${x^2} - 8x - 9 = 0$

$\Leftrightarrow {x^2} + x - 9x - 9 = 0$ $\Leftrightarrow {x^2} + x - 9x - 9 = 0$

$\Leftrightarrow x\left( {x + 1} \right) - 9\left( {x + 1} \right) = 0$ $\Leftrightarrow x\left( {x + 1} \right) - 9\left( {x + 1} \right) = 0$

$\Leftrightarrow \left( {x - 9} \right)\left( {x + 1} \right) = 0 \Leftrightarrow \left[ \begin{array}{l} x = 9\\ x = - 1 \end{array} \right.$ $\Leftrightarrow \left( {x - 9} \right)\left( {x + 1} \right) = 0 \Leftrightarrow \left[ \begin{array}{l} x = 9\\ x = - 1 \end{array} \right.$

Vậy S = {-1; 9}

b) $\sqrt {5x + 4} = x + 2$ $\sqrt {5x + 4} = x + 2$(1)

Điều kiện $5x + 4 \ge 0 \Leftrightarrow x \ge \frac{{ - 4}}{5}$ $5x + 4 \ge 0 \Leftrightarrow x \ge \frac{{ - 4}}{5}$

(1) $\Leftrightarrow \left\{ \begin{array}{l} x + 2 \ge 0\\ 5x + 4 = {\left( {x + 2} \right)^2} \end{array} \right. \Leftrightarrow \left\{ \begin{array}{l} x \ge - 2\\ 5x + 4 = {x^2} + 4x + 4 \end{array} \right.$ $\Leftrightarrow \left\{ \begin{array}{l} x + 2 \ge 0\\ 5x + 4 = {\left( {x + 2} \right)^2} \end{array} \right. \Leftrightarrow \left\{ \begin{array}{l} x \ge - 2\\ 5x + 4 = {x^2} + 4x + 4 \end{array} \right.$

$\Leftrightarrow \left\{ \begin{array}{l} x \ge - 2\\ {x^2} - x = 0 \end{array} \right. \Leftrightarrow \left\{ \begin{array}{l} x \ge - 2\\ \left[ \begin{array}{l} x = 0\\ x = 1 \end{array} \right.\left( {tm} \right) \end{array} \right.$ $\Leftrightarrow \left\{ \begin{array}{l} x \ge - 2\\ {x^2} - x = 0 \end{array} \right. \Leftrightarrow \left\{ \begin{array}{l} x \ge - 2\\ \left[ \begin{array}{l} x = 0\\ x = 1 \end{array} \right.\left( {tm} \right) \end{array} \right.$

Vậy S = {0; 1}

Bài 4:

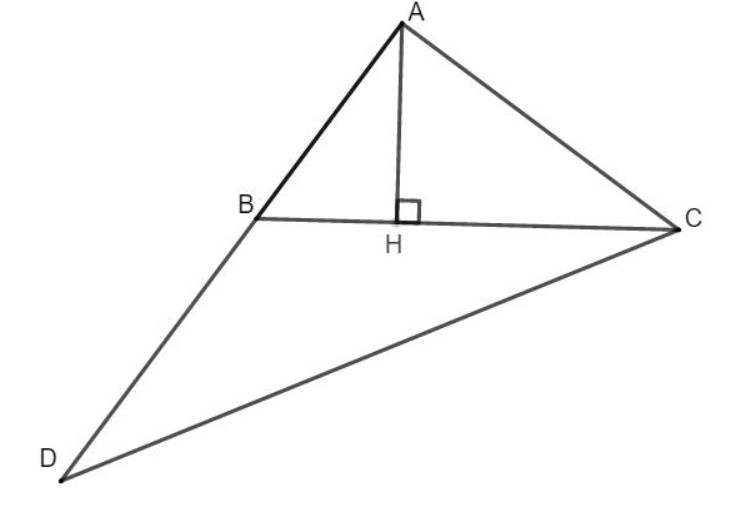
Đề thi giữa học kì 1 Toán 9 năm học 2020 - 2021 - Đề 2

a) Xét ∆ABC có:

$\left. \begin{array}{l} {{\mathop{\rm AB}\nolimits} ^2} + {{\mathop{\rm AC}\nolimits} ^2} = {6^2} + {8^2} = 100\\ {{\mathop{\rm BC}\nolimits} ^2} = {10^2} = 100 \end{array} \right\} \Rightarrow {{\mathop{\rm AB}\nolimits} ^2} + {{\mathop{\rm AC}\nolimits} ^2} = {{\mathop{\rm BC}\nolimits} ^2}$ $\left. \begin{array}{l} {{\mathop{\rm AB}\nolimits} ^2} + {{\mathop{\rm AC}\nolimits} ^2} = {6^2} + {8^2} = 100\\ {{\mathop{\rm BC}\nolimits} ^2} = {10^2} = 100 \end{array} \right\} \Rightarrow {{\mathop{\rm AB}\nolimits} ^2} + {{\mathop{\rm AC}\nolimits} ^2} = {{\mathop{\rm BC}\nolimits} ^2}$

⇒ABC vuông tại A (Pitago đảo)

b) Xét ∆ABC vuông tại A(cmt), có AH ⊥ BC:

+ ${{\mathop{\rm AB}\nolimits} ^2} = {\mathop{\rm BH}\nolimits} .BC$ ${{\mathop{\rm AB}\nolimits} ^2} = {\mathop{\rm BH}\nolimits} .BC$(hệ thức lượng trong tam giác vuông)

$\Rightarrow {\mathop{\rm BH}\nolimits} = \frac{{{{{\mathop{\rm AB}\nolimits} }^2}}}{{{\mathop{\rm BC}\nolimits} }} = \frac{{36}}{{100}} = \frac{9}{{25}}$ $\Rightarrow {\mathop{\rm BH}\nolimits} = \frac{{{{{\mathop{\rm AB}\nolimits} }^2}}}{{{\mathop{\rm BC}\nolimits} }} = \frac{{36}}{{100}} = \frac{9}{{25}}$(cm)

+ ${{\mathop{\rm AC}\nolimits} ^2} = {\mathop{\rm CH}\nolimits} .CB$ ${{\mathop{\rm AC}\nolimits} ^2} = {\mathop{\rm CH}\nolimits} .CB$(hệ thức lượng trong tam giác vuông)

$\Rightarrow {\mathop{\rm CH}\nolimits} = \frac{{{{{\mathop{\rm AC}\nolimits} }^2}}}{{{\mathop{\rm BC}\nolimits} }} = \frac{{64}}{{100}} = \frac{{16}}{{25}}$ $\Rightarrow {\mathop{\rm CH}\nolimits} = \frac{{{{{\mathop{\rm AC}\nolimits} }^2}}}{{{\mathop{\rm BC}\nolimits} }} = \frac{{64}}{{100}} = \frac{{16}}{{25}}$(cm)

+ ${{\mathop{\rm AH}\nolimits} ^2} = {\mathop{\rm BH}\nolimits} .HC$ ${{\mathop{\rm AH}\nolimits} ^2} = {\mathop{\rm BH}\nolimits} .HC$(hệ thức lượng trong tam giác vuông)

$\Rightarrow {{\mathop{\rm AB}\nolimits} ^2} = \frac{9}{{25}}.\frac{{16}}{{25}} \Rightarrow {\mathop{\rm AB}\nolimits} = \frac{{12}}{{25}}$ $\Rightarrow {{\mathop{\rm AB}\nolimits} ^2} = \frac{9}{{25}}.\frac{{16}}{{25}} \Rightarrow {\mathop{\rm AB}\nolimits} = \frac{{12}}{{25}}$(cm)

c) + Có AD = AB + BD = 6 + 10 = 16 (cm)

+ Xét ∆ADC vuông tại A có:

${{\mathop{\rm AD}\nolimits} ^2} + {{\mathop{\rm AC}\nolimits} ^2} = {{\mathop{\rm CD}\nolimits} ^2}$ ${{\mathop{\rm AD}\nolimits} ^2} + {{\mathop{\rm AC}\nolimits} ^2} = {{\mathop{\rm CD}\nolimits} ^2}$(Pitago)

$\Rightarrow {\mathop{\rm CD}\nolimits} = \sqrt {{{16}^2} + {8^2}} = 8\sqrt 5$ $\Rightarrow {\mathop{\rm CD}\nolimits} = \sqrt {{{16}^2} + {8^2}} = 8\sqrt 5$(cm)

+ Có AD.BC = 16.10 = 160

Và $\frac{{C{D^2}}}{2} = \frac{{320}}{2} = 160$ $\frac{{C{D^2}}}{2} = \frac{{320}}{2} = 160$

Vậy ${\mathop{\rm AD}\nolimits} .BC = \frac{{C{D^2}}}{2}$ ${\mathop{\rm AD}\nolimits} .BC = \frac{{C{D^2}}}{2}$

d) + ${{\mathop{\rm S}\nolimits} _{\Delta ABC}} = \frac{1}{2}{\mathop{\rm AB}\nolimits} .AC = \frac{1}{2}.6.8 = 24$ ${{\mathop{\rm S}\nolimits} _{\Delta ABC}} = \frac{1}{2}{\mathop{\rm AB}\nolimits} .AC = \frac{1}{2}.6.8 = 24$ (cm²)

+ ${{\mathop{\rm S}\nolimits} _{\Delta {\mathop{\rm ACD}\nolimits} }} = \frac{1}{2}{\mathop{\rm A}\nolimits} {\mathop{\rm D}\nolimits} .AC = \frac{1}{2}.16.8 = 64$ ${{\mathop{\rm S}\nolimits} _{\Delta {\mathop{\rm ACD}\nolimits} }} = \frac{1}{2}{\mathop{\rm A}\nolimits} {\mathop{\rm D}\nolimits} .AC = \frac{1}{2}.16.8 = 64$(cm²)

Vậy S_∆BCD = 64 – 24 = 40 (cm²)

...........................

Ngoài Bộ đề kiểm tra giữa kì 1 Toán 9 năm 2023 - 2024, các em học sinh có thể tham khảo thêm các đề thi giữa học kì 1 lớp 9 gồm các môn Toán, Văn, Hóa, Tiếng Anh...mà VnDoc sưu tầm và chọn lọc. Với những đề thi lớp 9 này sẽ giúp các em rèn luyện kỹ năng giải đề và làm bài tốt hơn. Chúc các em ôn thi tốt.

Chia sẻ, đánh giá bài viết

20.342