Giải Toán 10 Bài 6: Tích vô hướng của hai vectơ CD
Giải Toán 10 Bài 6 Chương 4 CD
Giải Toán 10 Bài 6: Tích vô hướng của hai vectơ CD được VnDoc.com sưu tầm và xin gửi tới bạn đọc. Bài viết hướng dẫn bạn đọc trả lời các câu hỏi trong SGK Toán 10 CD. Mời các bạn cùng theo dõi bài viết dưới đây.
Bài 1 trang 97 SGK Toán 10 CD
Nếu hai điểm M, N thỏa mãn 
\(\overrightarrow {MN} .\overrightarrow {NM} = - 4\) thì độ dài đoạn thẳng MN bằng bao nhiêu?
A. MN = 4
B. MN = 2
C. MN = 16
D. MN = 256
Lời giải
\(\overrightarrow {MN} .\overrightarrow {NM} = \left| {\overrightarrow {MN} } \right|.\left| {\overrightarrow {NM} } \right|.\cos (\overrightarrow {MN} ,\overrightarrow {NM} ) = M{N^2}.\cos {180^o} = - M{N^2}\)
Do đó: \(- M{N^2} = - 4 \Leftrightarrow MN = 2.\)
Bài 2 trang 98 SGK Toán 10 CD
Phát biểu nào sau đây là đúng?
A. Nếu \(\overrightarrow a ,\overrightarrow b\)khác \(\overrightarrow 0\)và \((\overrightarrow a ,\overrightarrow b ) < {90^o}\) thì \(\overrightarrow a .\overrightarrow b < 0\)
B. Nếu \(\overrightarrow a ,\overrightarrow b\)khác \(\overrightarrow 0\)và \((\overrightarrow a ,\overrightarrow b ) > {90^o}\) thì \(\overrightarrow a .\overrightarrow b > 0\)
C. Nếu \(\overrightarrow a ,\overrightarrow b\)khác \(\overrightarrow 0\)và \((\overrightarrow a ,\overrightarrow b ) < {90^o}\) thì \(\overrightarrow a .\overrightarrow b > 0\)
D. Nếu \(\overrightarrow a ,\overrightarrow b\)khác \(\overrightarrow 0\)và \((\overrightarrow a ,\overrightarrow b ) \ne {90^o}\) thì \(\overrightarrow a .\overrightarrow b < 0\)
Lời giải
Ta có: \(\overrightarrow a .\overrightarrow b = \left| {\overrightarrow a } \right|.\left| {\overrightarrow b } \right|.\cos (\overrightarrow a ,\overrightarrow b )\)
\(+)\ (\overrightarrow a ,\overrightarrow b ) < {90^o} \Rightarrow \cos (\overrightarrow a ,\overrightarrow b ) > 0 \Leftrightarrow \overrightarrow a .\overrightarrow b > 0\)
Vậy A đúng, C sai, D sai.
\(+)\ (\overrightarrow a ,\overrightarrow b ) > {90^o} \Rightarrow \cos (\overrightarrow a ,\overrightarrow b ) < 0 \Leftrightarrow \overrightarrow a .\overrightarrow b < 0\)
Vậy B sai.
Chọn A.
Bài 3 trang 98 SGK Toán 10 CD
Tính \(\overrightarrow a .\overrightarrow b\)trong mỗi trường hợp sau:
a) \(\left| {\overrightarrow a } \right| = 3,\;\left| {\overrightarrow b } \right| = 4,\;(\overrightarrow a ,\overrightarrow b ) = {30^o}\)
b) \(\left| {\overrightarrow a } \right| = 5,\;\left| {\overrightarrow b } \right| = 6,\;(\overrightarrow a ,\overrightarrow b ) = {120^o}\)
c) \(\left| {\overrightarrow a } \right| = 2,\;\left| {\overrightarrow b } \right| = 3,\;\overrightarrow a\)và \(\overrightarrow b\)cùng hướng.
d) \(\left| {\overrightarrow a } \right| = 2,\;\left| {\overrightarrow b } \right| = 3,\;\overrightarrow a\)và \(\overrightarrow b\)ngược hướng
Lời giải
a) \(\overrightarrow a .\overrightarrow b = 3.4.\cos {30^o} = 12.\frac{{\sqrt 3 }}{2} = 6\sqrt 3\)
b) \(\overrightarrow a .\overrightarrow b = 5.6.\cos {120^o} = 30.\left( { - \frac{1}{2}} \right) = - 15\)
c) \(\overrightarrow a\) và \(\overrightarrow b\) cùng hướng nên \((\overrightarrow a ,\overrightarrow b ) = {0^o}\)
\(\overrightarrow a .\overrightarrow b = 2.3.\cos {0^o} = 6.1 = 6\)
d) \(\overrightarrow a\) và \(\overrightarrow b\) ngược hướng nên \((\overrightarrow a ,\overrightarrow b ) = {180^o}\)
\(\overrightarrow a .\overrightarrow b = 2.3.\cos {180^o} = 6.( - 1) = - 6\)
Bài 4 trang 98 SGK Toán 10 CD
Cho hình vuông ABCD cạnh a. Tính các tích vô hướng sau:
a) \(\overrightarrow {AB} .\overrightarrow {AC}\)
b) \(\overrightarrow {AC} .\overrightarrow {BD}\)
Lời giải
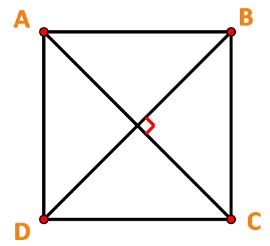
a) Ta có: \(AC = \sqrt {A{B^2} + A{D^2}} = \sqrt {2{a^2}} = a\sqrt 2\)
\(\Rightarrow \overrightarrow {AB} .\overrightarrow {AC} = a.a\sqrt 2 .\cos \widehat {BAC} = {a^2}\sqrt 2 \cos {45^o} = {a^2}.\)
b) Dễ thấy: \(AC \bot BD \Rightarrow (\overrightarrow {AC} ,\overrightarrow {BD} ) = {90^o}
\Rightarrow \overrightarrow {AC} .\overrightarrow {BD} = AC.BD.\cos {90^o} = AC.BD.0 = 0\).
Bài 5 trang 98 SGK Toán 10 CD
Cho tam giác ABC. Chứng minh: \(A{B^2} + \overrightarrow {AB} .\overrightarrow {BC} + \overrightarrow {AB} .\overrightarrow {CA} = 0\)
Lời giải
\(\begin{array}{l}A{B^2} + \overrightarrow {AB} .\overrightarrow {BC} + \overrightarrow {AB} .\overrightarrow {CA} = {\overrightarrow {AB} ^2} + \overrightarrow {AB} .\overrightarrow {BC} + \overrightarrow {AB} .\overrightarrow {CA} \\ = \overrightarrow {AB} (\overrightarrow {AB} + \overrightarrow {BC} + \overrightarrow {CA} ) = \overrightarrow {AB} (\overrightarrow {AC} + \overrightarrow {CA} ) = \overrightarrow {AB} .\overrightarrow 0 = 0.\end{array}\)
Bài 6 trang 98 SGK Toán 10 CD
Cho tam giác nhọn ABC, kẻ đường cao AH. Chứng minh rằng:
a) \(\overrightarrow {AB} .\overrightarrow {AH} = \overrightarrow {AC} .\overrightarrow {AH}\)
b) \(\overrightarrow {AB} .\overrightarrow {BC} = \overrightarrow {HB} .\overrightarrow {BC}\)
Lời giải
Ta có: \(AH \bot CB \Rightarrow (\overrightarrow {AH} ,\overrightarrow {CB} ) = {90^o} \Leftrightarrow \cos (\overrightarrow {AH} ,\overrightarrow {CB} ) = 0 \Leftrightarrow \overrightarrow {AH} .\overrightarrow {CB} = 0\)
a) \(\overrightarrow {AB} .\overrightarrow {AH} - \overrightarrow {AC} .\overrightarrow {AH} = (\overrightarrow {AB} - \overrightarrow {AC} ).\overrightarrow {AH} = \overrightarrow {CB} .\overrightarrow {AH} = 0\)
\(\Leftrightarrow \overrightarrow {AB} .\overrightarrow {AH} = \overrightarrow {AC} .\overrightarrow {AH}\)
b) \(\overrightarrow {AB} .\overrightarrow {BC} - \overrightarrow {HB} .\overrightarrow {BC} = (\overrightarrow {AB} - \overrightarrow {HB} ).\overrightarrow {BC} = (\overrightarrow {AB} + \overrightarrow {BH} ).\overrightarrow {BC} = \overrightarrow {AH} .\overrightarrow {BC} = 0\)
\(\Leftrightarrow \overrightarrow {AB} .\overrightarrow {BC} = \overrightarrow {HB} .\overrightarrow {BC}\)
Bài 7 trang 98 SGK Toán 10 CD
Một máy bay đang bay từ hướng đông sang hướng tây với tốc độ 700 km/h thì gặp luồng gió thổi từ hướng đông bắc sang hướng tây nam với tốc độ 40 km/h (Hình 68). Máy bay bị thay đổi vận tốc sau khi gặp gió thổi. Tìm tốc độ mới của máy bay (làm tròn kết quả đến hàng phần trăm theo đơn vị km/h.)
Lời giải
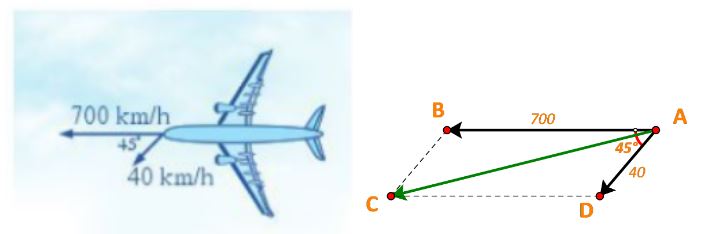
Vẽ vecto \(\overrightarrow {AB}\) là vecto vận tốc của máy bay, \(\overrightarrow {AD}\) là vecto vận tốc của gió.
Khi đó vecto vận tốc mới của máy bay là \(\overrightarrow {AB} + \overrightarrow {AD}\)
Dựng hình bình hành ABCD. Ta có: \(\overrightarrow {AB} + \overrightarrow {AD} = \overrightarrow {AC}\)
Áp dụng định lí cosin trong tam giác ABC, ta có:
\(A{C^2} = A{B^2} + B{C^2} - 2.AB.BC.\cos B\)
Mà \(AB = 700, BC = AD = 40, \widehat B = {135^o}\)
\(\begin{array}{l} \Rightarrow A{C^2} = {700^2} + {40^2} - 2.700.40.\cos {135^o} \approx 531197,98\\ \Leftrightarrow AC \approx 728,83\end{array}\)
Vậy tốc độ mới của máy bay là 728,83 km/h.
Bài 8 trang 98 SGK Toán 10 CD
Cho tam giác ABC có AB = 2, AC = 3,\(\widehat {BAC} = {60^o}\). Gọi M là trung điểm của đoạn thẳng BC. Điểm D thỏa mãn \(\overrightarrow {AD} = \frac{7}{{12}}\overrightarrow {AC}\).
a) Tính \(\overrightarrow {AB} .\overrightarrow {AC}\)
b) Biểu diễn \(\overrightarrow {AM} ,\overrightarrow {BD}\) theo \(\overrightarrow {AB} ,\overrightarrow {AC}\)
c) Chứng minh AM \bot BD.
Lời giải
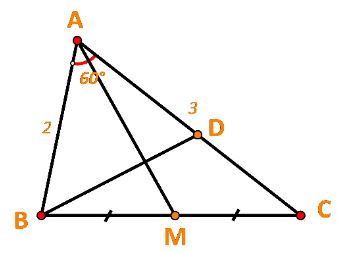
a) \(\overrightarrow {AB} .\overrightarrow {AC} = 2.3.\cos \widehat {BAC} = 6.\cos {60^o} = 3\)
b)
Ta có: \(\overrightarrow {AB} + \overrightarrow {AC} = 2\overrightarrow {AM}\)(do M là trung điểm của BC)
\(\Leftrightarrow \overrightarrow {AM} = \frac{1}{2}\overrightarrow {AB} + \frac{1}{2}\overrightarrow {AC}\)
+) \(\overrightarrow {BD} = \overrightarrow {AD} - \overrightarrow {AB} = \frac{7}{{12}}\overrightarrow {AC} - \overrightarrow {AB}\)
c) Ta có:
\(\begin{array}{l}\overrightarrow {AM} .\overrightarrow {BD} = \left( {\frac{1}{2}\overrightarrow {AB} + \frac{1}{2}\overrightarrow {AC} } \right)\left( {\frac{7}{{12}}\overrightarrow {AC} - \overrightarrow {AB} } \right)\\ = \frac{7}{{24}}\overrightarrow {AB} .\overrightarrow {AC} - \frac{1}{2}{\overrightarrow {AB} ^2} + \frac{7}{{24}}{\overrightarrow {AC} ^2} - \frac{1}{2}\overrightarrow {AC} .\overrightarrow {AB} \\ = - \frac{1}{2}A{B^2} + \frac{7}{{24}}A{C^2} - \frac{5}{{24}}\overrightarrow {AB} .\overrightarrow {AC} \\ = - \frac{1}{2}{.2^2} + \frac{7}{{24}}{.3^2} - \frac{5}{{24}}.3\\ = 0\end{array}\)
\(\Rightarrow AM \bot BD\)
VnDoc.com vừa gửi tới bạn đọc bài viết Giải Toán 10 Bài 6: Tích vô hướng của hai vectơ CD. Hi vọng qua đây bạn đọc có thêm nhiều tài liệu để học tập nhé. Mời các bạn cùng tham khảo thêm tài liệu học tập các môn Ngữ văn 10 CD, Tiếng Anh 10...
