Giải Toán 10 Bài 2: Tổng và hiệu của hai vectơ CTST

Giải Toán 10 Tập 1 sách CTST

1 283

Tổng và hiệu của hai vectơ

Bài 1 trang 93 SGK Toán 10 CTST
Bài 2 trang 93 SGK Toán 10 CTST
Bài 3 trang 93 SGK Toán 10 CTST
Bài 4 trang 93 SGK Toán 10 CTST
Bài 5 trang 93 SGK Toán 10 CTST
Bài 6 trang 93 SGK Toán 10 CTST
Bài 7 trang 93 SGK Toán 10 CTST
Bài 8 trang 93 SGK Toán 10 CTST

Giải Toán 10 Bài 2: Tổng và hiệu của hai vectơ CTST được VnDoc.com tổng hợp và xin gửi tới bạn đọc cùng tham khảo. Mời các bạn cùng theo dõi chi tiết bài viết dưới đây nhé.

Bài 1 trang 93 SGK Toán 10 CTST

Cho hình bình hành ABCD có O là giao điểm hai đường chéo và một điểm M tùy ý. Chứng minh rằng:

$a) \overrightarrow {BA} + \overrightarrow {DC} = \overrightarrow {0;}$

$b) \overrightarrow {MA} + \overrightarrow {MC} = \overrightarrow {MB} + \overrightarrow {MD}$

Gợi ý đáp án

a) ABCD là hình bình hành nên $\overrightarrow {DC} = \overrightarrow {AB}$

$\Rightarrow \overrightarrow {BA} + \overrightarrow {DC} = \overrightarrow {BA} + \overrightarrow {AB} = \overrightarrow {BB} = \overrightarrow 0$

$b) \overrightarrow {MA} + \overrightarrow {MC} = \left( {\overrightarrow {MB} + \overrightarrow {BA} } \right) + \left( {\overrightarrow {MD} + \overrightarrow {DC} } \right)$

$= \overrightarrow {MB} + \overrightarrow {MD} (Vì \overrightarrow {BA} + \overrightarrow {DC} = \overrightarrow {0} )$

Bài 2 trang 93 SGK Toán 10 CTST

Cho tứ giác ABCD, thực hiện cả phép cộng và trừ vectơ sau:

$a) \overrightarrow {AB} + \overrightarrow {BC} + \overrightarrow {CD} + \overrightarrow {DA};$

$b) \overrightarrow {AB} - \overrightarrow {AD}$

$c) \overrightarrow {CB} - \overrightarrow {CD}$ .

Gợi ý đáp án

a) $\overrightarrow {AB} + \overrightarrow {BC} + \overrightarrow {CD} + \overrightarrow {DA} = \left( {\overrightarrow {AB} + \overrightarrow {BC} } \right) + \left( {\overrightarrow {CD} + \overrightarrow {DA} } \right)$

$= \overrightarrow {AC} + \overrightarrow {CA} = \overrightarrow {AA} = \overrightarrow 0$

$b) \overrightarrow {AB} - \overrightarrow {AD} = \overrightarrow {AB} + \overrightarrow {DA} = \overrightarrow {DA} + \overrightarrow {AB} = \overrightarrow {DB}$

$c) \overrightarrow {CB} - \overrightarrow {CD} = \overrightarrow {CB} + \overrightarrow {DC} = \overrightarrow {DC} + \overrightarrow {CB} = \overrightarrow {DB}$

Bài 3 trang 93 SGK Toán 10 CTST

Cho tam giác đều ABC cạnh bằng a. Tính độ dài các vectơ:

$a) \overrightarrow {BA} + \overrightarrow {AC} ;$

$b) \overrightarrow {AB} + \overrightarrow {AC} ;$

$c) \overrightarrow {BA} - \overrightarrow {BC} .$

Gợi ý đáp án

$a) \overrightarrow {BA} + \overrightarrow {AC} = \overrightarrow {BC} \Rightarrow \left| {\overrightarrow {BC} } \right| = BC = a$

b) Dựng hình bình hành ABDC, giao điểm của hai đường chéo là O ta có:

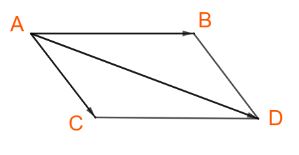
Giải Toán 10 Bài 2 CTST

$\overrightarrow {AB} + \overrightarrow {AC} = \overrightarrow {AD}$

$AD = 2AO = 2\sqrt {A{B^2} - B{O^2}} = 2\sqrt {{a^2} - {{\left( {\frac{a}{2}} \right)}^2}} = a\sqrt 3$

$\Rightarrow \left| {\overrightarrow {AB} + \overrightarrow {AC} } \right| = \left| {\overrightarrow {AD} } \right| = AD = a\sqrt 3$

$c) \overrightarrow {BA} - \overrightarrow {BC} = \overrightarrow {BA} + \overrightarrow {CB} = \overrightarrow {CB} + \overrightarrow {BA} = \overrightarrow {CA}$

$\Rightarrow \left| {\overrightarrow {BA} - \overrightarrow {BC} } \right| = \left| {\overrightarrow {CA} } \right| = CA = a$

Bài 4 trang 93 SGK Toán 10 CTST

Cho hình bình hành ABCD có O là giao điểm hai đường chéo. Chứng minh rằng:

$a) \overrightarrow {OA} - \overrightarrow {OB} = \overrightarrow {OD} - \overrightarrow {OC;}$

$b) \overrightarrow {OA} - \overrightarrow {OB} + \overrightarrow {DC} = \overrightarrow 0$

Gợi ý đáp án

$a) \overrightarrow {OA} - \overrightarrow {OB} = \overrightarrow {BA}$

$\overrightarrow {OD} - \overrightarrow {OC} = \overrightarrow {CD}$

Do ABCD là hình bình hành nên $\overrightarrow {BA} = \overrightarrow {CD}$

Suy ra, $\overrightarrow {OA} - \overrightarrow {OB} = \overrightarrow {OD} - \overrightarrow {OC}$

$b) \overrightarrow {OA} - \overrightarrow {OB} + \overrightarrow {DC} = (\overrightarrow {OD} - \overrightarrow {OC}) + \overrightarrow {DC} \\= \overrightarrow {CD} + \overrightarrow {DC} = \overrightarrow {CC} = \overrightarrow 0$

Bài 5 trang 93 SGK Toán 10 CTST

Cho ba lực $\overrightarrow {{F_1}} = \overrightarrow {MA} ,\overrightarrow {{F_2}} = \overrightarrow {MB} và \overrightarrow {{F_3}} = \overrightarrow {MC}$ cùng tác động vào một vật tại điểm M và vật đứng yên. Cho biết cường độ của $\overrightarrow {{F_1}} ,\overrightarrow {{F_2}}$ đều là 10 N và $\widehat {AMB} = 90^\circ$ Tìm độ lớn của lực $\overrightarrow {{F_3}} .$

Gợi ý đáp án

Ba lực $\overrightarrow {{F_1}} ,\overrightarrow {{F_2}} ,\overrightarrow {{F_3}}$ cùng tác dụng vào M và vật đứng yên nên hợp lực của chúng có giá trị bằng không, hay: $\overrightarrow {{F_1}} + \overrightarrow {{F_2}} + \overrightarrow {{F_3}} = \overrightarrow {MA} + \overrightarrow {MB} + \overrightarrow {MC} = \overrightarrow 0$

Dựng hình bình hành MADB, khi đó: $\overrightarrow {MA} + \overrightarrow {MB}= \overrightarrow {MD}$

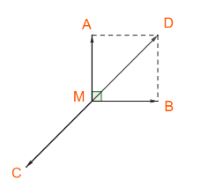
Giải Toán 10 Bài 2 CTST

$\Rightarrow \overrightarrow {MD} + \overrightarrow {MC} = \overrightarrow {0}$

$\Rightarrow \overrightarrow {MD}, \overrightarrow {MC}$ là hai vecto đối nhau

$\Rightarrow MD =MC$

Xét hình bình hành MADB, ta có:

AM=AB và $\widehat {AMB} = 90^\circ$

$\Rightarrow$ MADB là hình vuông, cạnh AB=10

$\Rightarrow MC = MD = AB. \sqrt{2} = 10\sqrt{2}$

Vậy độ lớn của lực $\overrightarrow {{F_3}}$ là $\left| {\overrightarrow {{F_3}} } \right| = \left| {\overrightarrow {MC} } \right| = MC = 10\sqrt 2 (N)$

Bài 6 trang 93 SGK Toán 10 CTST

Khi máy bay nghiêng cánh một góc $\alpha ,$ lực $\overrightarrow F$ của không khí tác động vuông góc với cánh và bằng tổng của lực nâng $\overrightarrow {{F_1}}$ và lực cản $\overrightarrow {{F_2}}$ (Hình 16). Cho biết $\alpha = 30^\circ$ và $\left| {\overrightarrow F } \right| = a.$ Tính $\left| {\overrightarrow {{F_1}} } \right|$ và $\left| {\overrightarrow {{F_2}} } \right|$ theo a.

Gợi ý đáp án

Kí hiệu các điểm như hình dưới.

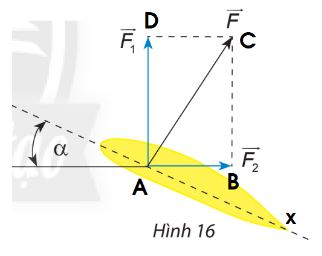
Giải Toán 10 Bài 2 CTST

Khi đó các lực $\overrightarrow F ,\overrightarrow {{F_1}} ,\overrightarrow {{F_2}} lần lượt là \overrightarrow {AC} ,\overrightarrow {AD} ,\overrightarrow {AB}$

$\alpha = \widehat {{\rm{BAx}}} = 30^\circ \Rightarrow \widehat {CAB} = 60^\circ$

$AB = AC.c{\rm{os}}\widehat {CAB} = a.c{\rm{os60}}^\circ {\rm{ = }}\frac{a}{2} \Rightarrow \left| {\overrightarrow {{F_2}} } \right| = \left| {\overrightarrow {AB} } \right| = \frac{a}{2}$

$AD = BC = AC.\sin \widehat {CAB} = a.\sin 60^\circ = \frac{{a\sqrt 3 }}{2} \Rightarrow \left| {\overrightarrow {{F_1}} } \right| = \left| {\overrightarrow {AD} } \right| = AD = \frac{{a\sqrt 3 }}{2}$

Vậy $\left| {\overrightarrow {{F_1}} } \right| = \frac{{a\sqrt 3 }}{2};\left| {\overrightarrow {{F_2}} } \right| = \frac{a}{2}$

Bài 7 trang 93 SGK Toán 10 CTST

Cho hình vuông ABCD có cạnh bằng a và ba điểm G, H, K thỏa mãn $\overrightarrow {KA} + \overrightarrow {KC} = \overrightarrow 0 ;\overrightarrow {GA} + \overrightarrow {GB} + \overrightarrow {GC} = \overrightarrow 0 ;\overrightarrow {HA} + \overrightarrow {HD} + \overrightarrow {HC} = \overrightarrow 0$ . Tính độ dài các vectơ $\overrightarrow {KA} ,\overrightarrow {GH} ,\overrightarrow {AG} .$

Gợi ý đáp án

Ta có $AC = AB\sqrt 2 = a\sqrt 2$

$+) \overrightarrow {KA} + \overrightarrow {KC} = \overrightarrow 0 ,$

Suy ra K là trung điểm $AC \Rightarrow AK = \frac{1}{2}.a\sqrt 2 = \frac{{a\sqrt 2 }}{2}$

$+) \overrightarrow {HA} + \overrightarrow {HD} + \overrightarrow {HC} = \overrightarrow 0$ , suy ra H là trọng tâm của tam giác ADC

$\Rightarrow DH = \frac{2}{3}DK = \frac{1}{3}DB (1)$

$+) \overrightarrow {GA} + \overrightarrow {GB} + \overrightarrow {GC} = \overrightarrow 0$ , suy ra G là trọng tâm của tam giác ABC

$\Rightarrow BG = \frac{2}{3}BK = \frac{1}{3}BD (2)$

$(1,2) \Rightarrow HG = \frac{1}{3}BD=\frac{{a\sqrt 2 }}{3}$

Mà $KG = KH = \frac{1}{2}HG= \frac{{a\sqrt 2 }}{6} (2)$

$\Rightarrow AG = \sqrt {A{K^2} + G{K^2}} = \sqrt {{{\left( {\frac{{a\sqrt 2 }}{2}} \right)}^2} + {{\left( {\frac{{a\sqrt 2 }}{6}} \right)}^2}} = \frac{{a\sqrt 5 }}{3}$

$\Rightarrow \left| {\overrightarrow {AG} } \right| = \frac{{a\sqrt 5 }}{3}$

Vậy $\left|\overrightarrow {KA}\right| =\frac{{a\sqrt 2 }}{2} ,\left|\overrightarrow {GH}\right|=\frac{{a\sqrt 2 }}{3} ,\left|\overrightarrow {AG}\right|=\frac{{a\sqrt 5 }}{3} .$

Bài 8 trang 93 SGK Toán 10 CTST

Một con tàu có vectơ vận tốc chỉ theo hướng nam, vận tốc của dòng nước là một vectơ theo hướng đông như hình 17. Tính độ dài vectơ tổng của hai vectơ nói trên.

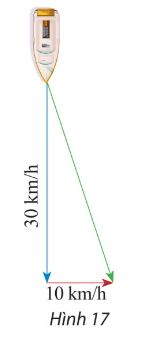
Giải Toán 10 Bài 2 CTST

Gợi ý đáp án

Gọi vecto vận tốc của tàu là $\overrightarrow {AB}$ , vecto vận tốc của dòng nước là vecto $\overrightarrow {BC}$

Giải Toán 10 Bài 2 CTST

Ta có vectơ tổng là $\overrightarrow F = \overrightarrow {AB} + \overrightarrow {BC} = \overrightarrow {AC}$

Độ dài vectơ tổng là $\left| {\overrightarrow F } \right| = \left| {\overrightarrow {AC} } \right| = AC = \sqrt {A{B^2} + B{C^2}} = \sqrt {{{30}^2} + {{10}^2}} = 10\sqrt {10} (km/h)$

Vậy độ dài vecto tổng là $10\sqrt {10}$ (km/h).

Trên đây VnDoc.com vừa gửi tới bạn đọc bài viết Giải Toán 10 Bài 2: Tổng và hiệu của hai vectơ CTST. Mong rằng qua bài viết này bạn đọc có thêm nhiều tài liệu để học tập tốt hơn môn Toán 10 CTST. Mời các bạn cùng tham khảo thêm tài liệu học tập môn Ngữ văn 10 CTST...

Đánh giá bài viết

1 283

Bài trước

Bài sau