Giải Toán 12 trang 60 tập 1 Chân trời sáng tạo
Giải Toán 12 trang 60 Tập 1
Giải Toán 12 trang 60 Tập 1 hướng dẫn giải chi tiết cho các câu hỏi và bài tập trong SGK Toán 12 Chân trời sáng tạo tập 1 trang 60.
Thực hành 2 trang 60 SGK Toán 12 tập 1 Chân trời
Cho ba vectơ 
\(\overrightarrow m = ( - 5;4;9), \overrightarrow n = (2; - 7;0), \overrightarrow p = (6;3; - 4)\).
a) Tính \(\overrightarrow{m}.\overrightarrow{n},\overrightarrow{m}.\overrightarrow{p\ }\)
b) Tính \(|\overrightarrow{m}|,|\overrightarrow{n}|,\cos(\overrightarrow{m},\overrightarrow{n})\)
c) Cho \(\overrightarrow{q}=(1;-2;0)\). Vectơ \(\overrightarrow{q\ }\) có vuông góc với \(\overrightarrow{p\ }\) không?
Hướng dẫn giải:
a) Ta có: \(\overrightarrow{m}.\overrightarrow{n\ }\) = (- 5) . 2 + 4. (- 7) + 9 . 0 = - 38
\(\overrightarrow{m}.\overrightarrow{p\ }\) = (- 5) . 6 + 4 . 3 + 9 . (- 4) = - 54
b) \(|\overrightarrow{m}|=\sqrt{\left(-5\right)^2+4^2+9^2}=\sqrt{122}\)
\(|\overrightarrow{n}|=\sqrt{2^2+\left(-7\right)^2+0^2}=\sqrt{53}\)
\(\cos(\overrightarrow{m},\overrightarrow{n})=\frac{\overrightarrow{m}.\overrightarrow{n}}{\left|\overrightarrow{m}\right|.\left|\overrightarrow{n}\right|}=\frac{-38}{\sqrt{122}.\sqrt{53}}\approx-0,468\)
c) Ta có: \(\overrightarrow{q}.\overrightarrow{p\ }\) = 0
Vậy vectơ \(\overrightarrow{q\ }\) có vuông góc với \(\overrightarrow{p\ }\)
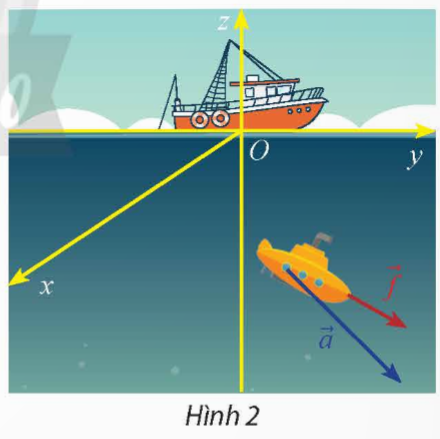
Vận dụng 2 trang 60 SGK Toán 12 tập 1 Chân trời
Một thiết bị thăm dò đáy biển (Hình 2) được đẩy bởi một lực \(\overrightarrow{f}=(5;4;-2)\) (đơn vị: N) giúp thiết bị thực hiện độ dời \(\overrightarrow a = (70;20; - 40)\) (đơn vị: m). Tính công sinh bởi lực \(\overrightarrow{f\ }\)
Hướng dẫn giải:
Công sinh bởi lực \(\overrightarrow{f\ }\) khi thực hiện độ dời \(\overrightarrow{a\ }\) là:
\(A=\overrightarrow{f\ }.\overrightarrow{a}=5.70+4.20+\left(-2\right).\left(-40\right)=510\) (N)
Hoạt động 3 trang 60 SGK Toán 12 tập 1 Chân trời
Cho hai điểm \(A({x_A};{y_A};{z_A}),B({x_B};{y_B};{z_B})\). Từ biểu thức \(\overrightarrow{AB}=\overrightarrow{OB}-\overrightarrow{OA}\), tìm toạ độ của vectơ \(\overrightarrow{AB}\) theo toạ độ hai điểm A, B.
Hướng dẫn giải:
\(\overrightarrow{OA}=\left(x_A;y_A;z_A\right);\ \overrightarrow{OB}=\left(x_B;y_B;z_B\right)\)
Vậy \(\overrightarrow{AB}=\overrightarrow{OB}-\overrightarrow{OA}=\left(x_B-x_A;y_B-y_A;z_B-z_A\right)\)
-----------------------------------------------
---> Xem thêm: Giải Toán 12 trang 61 tập 1 Chân trời sáng tạo
Lời giải Toán 12 trang 60 Tập 1 Chân trời sáng tạo với các câu hỏi nằm trong Bài 3: Biểu thức toạ độ của các phép toán vectơ, được VnDoc biên soạn và đăng tải!
